10-1 Helium Induces Grain Boundary Fracture of Iron

－Finding a Universal Law of Grain Boundary Strength Loss Using a Supercomputer－

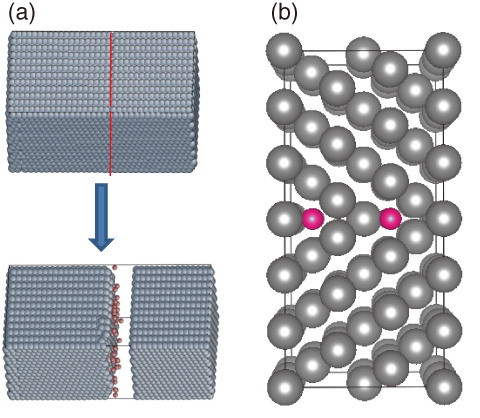
Fig.10-2　Calculation method for GB cohesive energy

Fig.10-2　Calculation method for GB cohesive energy

GB cohesive energy is defined as the total energy increase per unit area after separating the system at the GB, as shown in (a). The GB used for the present study is symmetrical at the boundary, as seen in (b), being called the symmetrical tilt GB.

 

Fig.10-3　The universal relationship between GB cohesive energy and He concentration

Fig.10-3　The universal relationship between GB cohesive energy and He concentration

GB cohesive energy decreases as He is accumulated. The above value is normalized to the cohesive energy without He accumulation.

Reduced activation ferritic steels are being considered as structural materials of future nuclear fusion reactors. However, it is expected that these steels will become brittle after long-term exposure to a fusion reactor’s environment. This may be caused by a decrease in the strength of the grain boundaries (GBs), which are surfaces between two adjacent grains. We suspect that loss of the strength may be caused by helium (He) accumulation at the GBs, which is generated by nuclear transmutation reactions under fast neutron irradiation.

He is accumulated either in the form of bubbles or in an atomic form without forming bubbles. The former occurs under high temperatures and can be observed by electron microscopes. The latter is supposed to occur at low temperatures, but it is difficult to determine the locations or the amount of He involved using experimental means; therefore, the relationship between the GB cohesive energy and the amount of accumulated He is not easily known. For this reason, we particularly focused on the application of computational methodology to He embrittlement without bubble formation under low temperatures.

In the present study, we defined GB cohesive energy as the total system energy increase due to ideal fracture (Fig.10-2) at the GB divided by its area. If this total energy increases, the cohesive energy is positive and a fracture is not expected; otherwise, the fracture is more likely to occur. We evaluated this energy using an atomistic modeling method.

Note that there are plenty types of GBs. In the current study, symmetrical tilt GBs (Fig.10-2(b)), which are suitable for our atomistic modeling, were considered. We searched for He accumulation positions for various GBs and found that these varied with the type of GBs. We artificially inserted He atoms at these positions ( in Fig.10-2(b)) and calculated GB cohesive energy as a function of He concentration on the GB. The results for many types of GBs are shown in Fig.10-3. It is known that GB cohesive energy is significantly influenced by He formation energy, which is GB-dependent; however, we found that GB cohesive energy was not dependent on the type of GBs, and was almost uniquely determined by He concentration. This result is critical, because it suggests that investigations on a single GB are sufficient to gauge GB embrittlement caused by He accumulation. As there exist an infinite number of GBs, the benefit gained by this result could significantly increase the efficiency of these types of investigations for other materials.

Reference
Suzudo, T. et al., Atomistic Modeling of He Embrittlement at Grain Boundaries of α-Fe: a Common Feature over Different Grain Boundaries, Modelling and Simulation in Materials Science and Engineering, vol.21, no.8, 2013, p.085013-1-085013-8.

 |