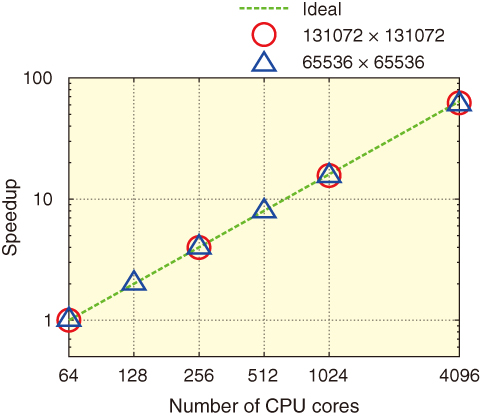
Fig.10-7 Strong scaling plot
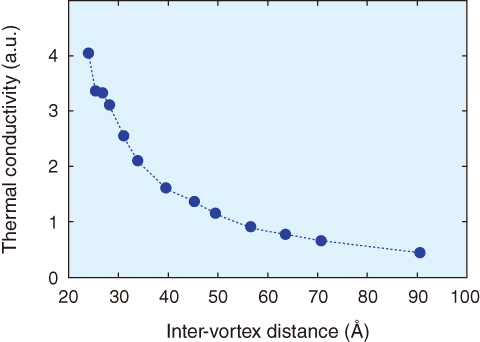
Fig.10-8 Magnetic field dependence of the thermal conductivity in a superconductor
Superconductivity is one of the most remarkable phenomena in condensed matter systems. A typical behavior is the sudden disappearance of electrical resistance below the superconducting transition temperature. Presently, in the nuclear R&D field, potential applications of the superconductors are being intensively studied. In fact, a superconducting coil will be indispensable for a nuclear fusion reactor. The superconductors are also applicable for the device in the strong radiation such as that with the space probes.
Nano-superconductors are the candidates for replacing the silicon-based semi-conductors. There are various kinds of applications such as the micro SQUID and single photon detectors. Thus, the simulation technique dealing with quantum phenomena in superconductors is very important. In the simulations for superconductors, the superconducting order parameter has to be self-consistently determined by solving the eigenvalue problems of the Hamiltonian matrix. However, the size of the matrix for the nano-superconductors with real-size scale is too huge to calculate with the previous numerical methods. Thus, we developed the simulation technique with the high-performance eigenvalue solver specialized in the massively-parallel supercomputers.
By using the massively-parallel Chebyshev polynomial expansion method for the self-consistently calculations and Sakurai-Sugiura method for calculating physical quantities, we performed the simulations for superconductors with the largest size in the world. Our method has the high-performance even with 4096 CPU cores as shown in Fig.10-7. With the use of our method, we calculated the magnetic field dependence of the thermal conductivity, which is difficult to calculate in the previous methods (Fig.10-8).
We revealed that Sakurai-Sugiura method, that the eigenvalues in a given domain can be obtained very fast, is efficient for calculating the physical quantities in the nano-superconductors. Our high-performance simulations code might be efficient even in the exa-scale supercomputers.
By combining our code with supercomputers, the real-size scale simulations for superconducting devices can be performed and novel functional materials and devices can be constructed in the future.
The present study was partly supported by Japan Society for the Promotion of Science (JSPS) KAKENHI Grant-in-Aid for Scientific Research (B) (No.24340079).