4-7　Predicting Mechanical Properties of Alloys

－Computational Approach for Modeling the Strengthening/Softening Mechanism－

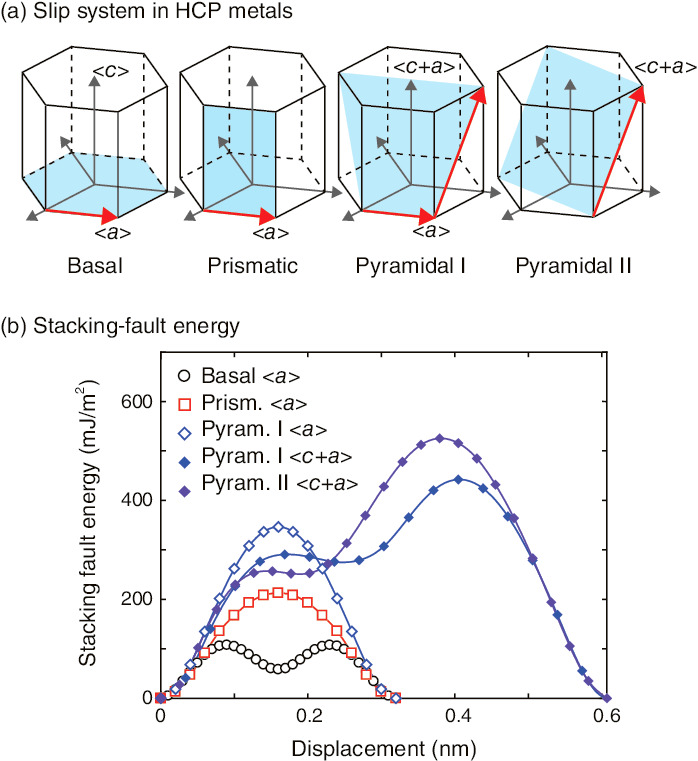
Fig.4-15 Crystallographic structure of HCP metals:

Fig.4-15 Crystallographic structure of HCP metals: (a) slip plane and (b) stacking-fault energy

HCP metals have various slip planes. Potential slip directions are shown by red arrows for each plane. The energy differences, defined as stacking-fault energies, are shown in (b). Basal slip is expected to be the primary slip system.

 

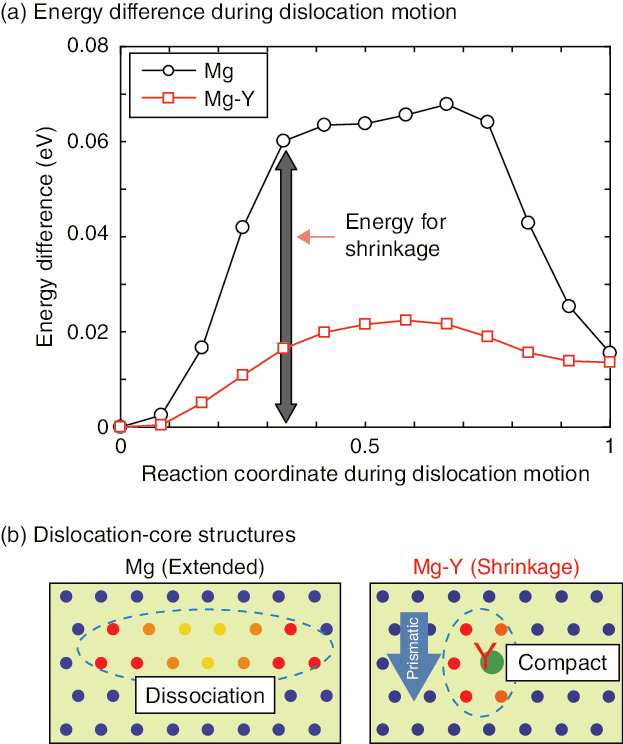
Fig.4-16 Effect of alloying elements upon dislocation motion

Fig.4-16 Effect of alloying elements upon dislocation motion

(a) Energy difference during the motion of dislocations in the prismatic plane and (b) dislocation-core structures in pure Mg and Mg-Y alloys. The energy barrier for prismatic slip is reduced by the Y solution due to the strong electronic interaction.

 

Hexagonal close-packed (HCP) metals such as magnesium (Mg), titaniun (Ti), and zirconium (Zr) exhibit excellent material properties including a lightweight structure, heat resistance, and corrosion resistance. Widespread application, however, has been limited because of a key weakness stemming from their HCP crystal structure. Unlike iron or aluminum, HCP metals have various slip planes, causing anisotropic plastic deformation. This makes it more important to improve the formability through plastic working. Solution strengthening is a well-known approach to tailoring the mechanical properties of structural alloys. Ultimately, the properties of the dislocation/solute interaction are rooted in the electronic structure of the alloy. A computational approach to predicting the effect of alloying upon mechanical properties plays a crucial role in non-empirical alloy design.

Here, we introduce an electronic-structure-based approach to understand the dislocation-solute interaction. Fig.4-15 shows possible slip planes in HCP metals and energy difference along a red arrow. The energy difference is defined as a stacking-fault energy that determines the dislocation-core structure and motion. Fig.4-15(b) shows that energy along <a> in the basal plane is extremely low, while those values in the non-basal planes are high. Reducing energy difference in the prismatic plane is a potential approach to improving plastic anisotropy.

Subsequently, we have constructed a dislocation-quadrupole model in a periodic cell and evaluated energy difference during motion in the prismatic plane, as shown in Fig.4-16(a). yttrium (Y) solute definitely reduces the energy barrier for dislocation motion in the prismatic plane. The transition states of dislocation-core structure during the motion are shown in Fig.4-16(b). Interestingly, the dissociated dislocation in pure Mg tends to shrink due to the strong electronic interaction with Y, which promotes dislocation motion on non-basal planes and reduces plastic anisotropy. Computational models based on atomistic simulations are expected to be an effective approach for new-alloy design.

Reference
Tsuru, T. et al., Effect of Solute Atoms on Dislocation Motion in Mg: An Electronic Structure Perspective, Scientific Reports, vol.5, 2015, p.8793-1-8793-8.

 |