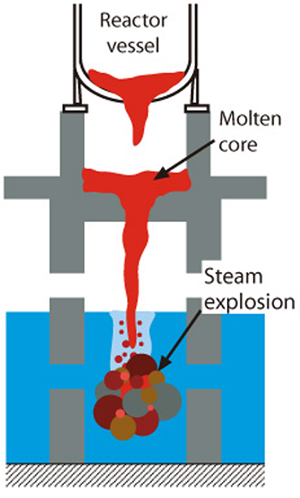
Fig.5-14 A steam explosion during a severe accident of a light water reactor
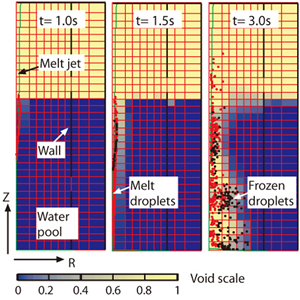
Fig.5-15 Simulation of the premixing process by the JASMINE code
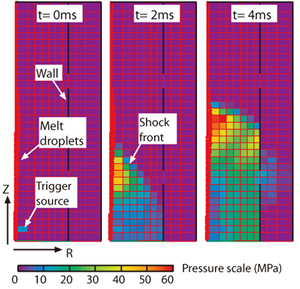
Fig.5-16 Simulation of the explosion process by the JASMINE code
A steam explosion, an extremely rapid production of high pressure steam, occurs when a hot liquid (e.g. molten metal) drops into water. It may occur in severe accidents of nuclear power plants (involving core melt down; e.g. Three Mile Island accident in U.S.) when the molten core drops into water. (Fig.5-14) If the shock load by the explosion breaks the containment vessel, radioactive material may leak into the environment. Thus, the steam explosion has been regarded as a cause of a large release and has been studied intensively for a long time. It is now accepted that the frequency of a steam explosion is very low so that its contribution to the public risk (product of the frequency and radiological consequences) is small in the probabilistic safety assessment (PSA). However, the uncertainty of the steam explosion risk is a remained issue.
Although much research has been done to clarify the mechanism and the load of steam explosions, such knowledge could not be directly applied to plant scale assessment due to differences in scale and material between experiments and real plants. We needed something that bridges this gap, and so developed a steam explosion simulation code, JASMINE, and applied it for the evaluation of the containment failure probability which is necessary for the risk analysis.
Fig.5-15 and Fig.5-16 are example calculations by JASMINE. In a steam explosion, the molten core breaks-up in the water and mixes with water and steam," premixing" (Fig.5-15), which is followed by the explosion where rapid steam generation causes a high pressure in a few milliseconds (Fig.5-16). JASMINE models such phenomena by an extended multiphase flow simulation method, conventionally used for the analysis of reactor cooling system and so on.
We applied JASMINE in a probabilistic framework to evaluate the containment failure probability. We made calculations for sets of initial/boundary conditions and model parameters sampled by reflecting their probability distributions, and obtained the probability distributions of the loads. The results were compared with fragility curves of the containment vessel to evaluate the containment failure probabilities. The containment failure probabilities obtained by this method were in the range 0.01~0.1 (mean, per explosion triggering), in agreement with the values used in PSAs in the past. To get the frequency of radioactive release by a steam explosion, this result should be multiplied by the core damage frequency and the probability of the steam explosion occurrence after core damage.
This method, combining a mechanistic simulation and a probabilistic framework, gives a clear explanation for the basis of PSA results, and helps the clarification of uncertainty sources.
This work includes a part of a contract research project sponsored by Ministry of Education, Culture, Sports, Science and Technology (MEXT).