
Fig.3-2 Shape evolution and fission for two fermium isotopes, 254Fm and 258Fm
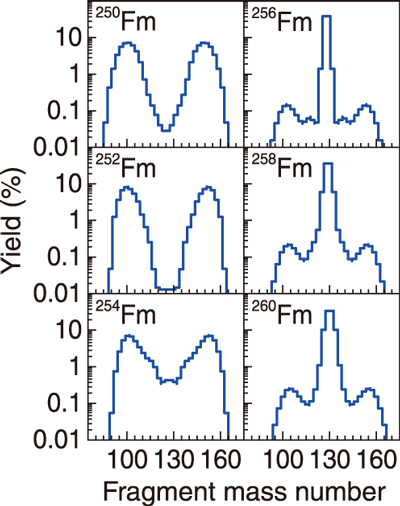
Fig.3-3 Calculated fission fragment mass distributions
In the approximately 80 years since the discovery of nuclear fission, the phenomenon has been applied to atomic power reactors. Neutrons from research reactors are used for cancer therapy and fundamental science research. The fission of uranium produces two fragments with large and small mass numbers, called as mass-asymmetric fission. Due to its complexity, the fission mechanism has yet not been fully understood, remaining as a challenging subject in nuclear physics. Nuclear fission also has a large impact on other fields of science, such as super-heavy element research, noticed by the discovery of element 113, led by RIKEN team. The existing limit of the heaviest element is determined by its stability against fission, which can be predicted by understanding the nuclear-shape evolution in fission over the wide range of nuclides toward heavy elements.
Approximately 40 years ago, surprising results regarding the fission of fermium isotopes (Fm, atomic number 100) was reported. Whereas the lighter Fm isotope shows a mass-asymmetric fission, the heavier nuclide 258Fm exhibits a mass-symmetric fission with a prominent peak structure. This unique fission is expected to dominate in the super-heavy element region, thus influencing stability against fission. Understanding the fission of fermium region makes an important step in this field; however, a quantitative description of this phenomenon has not yet been achieved.
The model presented here successfully explained the mechanism of this phenomenon. The potential energy of nuclides 254Fm and 258Fm is shown in Fig.3-2, in which the nuclear shape is shown to evolve with time by following the local minima. For 254Fm, the nucleus was trapped in the intermediate local minimum after overcoming the first saddle point before fissioning by passing over the saddle point B. On the contrary, 258Fm fissions by surmounting the saddle point A, without staying in the local minimum. A significant change was thus demonstrated: asymmetric fission to sharp symmetric fission, as shown in Fig.3-3. The nucleus has two saddle points, A and B, at a similar height. By reaching to the heavier isotope 258Fm, the system can eventually undergo fission by passing through saddle point A. The mechanism is similar to that of a seesaw, where the slope can turn over quickly by a subtle balance or imbalance of the weights: a small change in the mass of a fissioning nucleus can guide the fission in a different direction. The fission mode inherent in 258Fm would be a common feature for super-heavy nuclei. Thus, this theoretical approach can be considered as a first step for deeper understanding of the heaviest nuclei.
The results were obtained from a student involved with the summer student program of JAEA. For the improvement of the theoretical model, the experimental data taken at the JAEA tandem facility in Tokai were used.